Some of his most famous results are
- Partition formula (Hardy-Ramanujan-Rademacher asymptotic formula).
This formula helped in establishing the fame of Ramanujan in the world of mathematics. He along with Hardy discovered and proved an asymptotic formula for the well known partition function which helps to approximate the function for large enough numbers. This function also has a lot of applications both within number theory and outside.
- 1+2+3+…= -1/12
Before saying anything, it should be informed that this identity is not true in the general sense. But this has a deep meaning which is why this is so famous. The constant on the right is extremely famous for being the analytical continuation of the Riemann zeta function at -1. Ramanujan devised an unique method to evaluate the Zeta function for negative integers. This technique is called the Ramanujan summation.
- Ramanujan congruences.
Ramanujan independently discovered three congruences related to the partition function. These are perhaps the most beautiful results given by him. These congruences were later proved by Hardy and generalised by later mathematicians.
One such congruence is p(5n+4) = 0(mod 5).
- Ramanujan master theorem.
This theorem is one of the most applied results of Ramanujan. This is used to evaluate the Mellin transforms of several complex valued functions. This has been generalised and now this is used in Quantum mechanics through Feynmann diagrams.
- Theta functions and their results.
Ramanujan greatly studied a group of functions which he named as the theta functions. These functions have been found to have a large number of properties which are applicable in advanced physics and mathematics. For example, these functions are used in the working of nuclear reactors.
- Mock theta functions.
Another class of functions discovered and studied by him. These are even more advanced and applicable than theta functions. Ramanujan described them in his last letter to Hardy. These functions are extremely useful in Quantum field theory and string theory. These functions are used for predicting the entropy of black holes.
- Rogers Ramanujan identities.
Ramanujan discovered these identities while studying continued fractions. These identities were earlier discovered by Rogers. These identities also have beautiful properties and applications.
- Ramanujan’s approximation for π.
The most efficient approximation for π till date. Only the first term of this series can approximate π upto eight places after the decimal point which is a fair approximation for most practical calculations. Modern computers use this technique to approximate π upto trillions of decimal places.
- Ramanujan’s results on highly composite numbers.
Ramanujan got his BSc degree after writing his paper entitled “ Highly composite numbers.” He discovered these numbers and studied them and as a result discovered highly original results in the topic.
- Generalization of Bertrand’s postulate.
One of the three mathematicians who proved the Bertrand’s postulate was Ramanujan. His short proof involved advanced properties of the gamma function and in this proof, he generalized the result which is the best generalization till date. Later on his methods of generalization were used by several mathematicians like Sondow who first defined and studied the Ramanujan primes.
An anecdote:
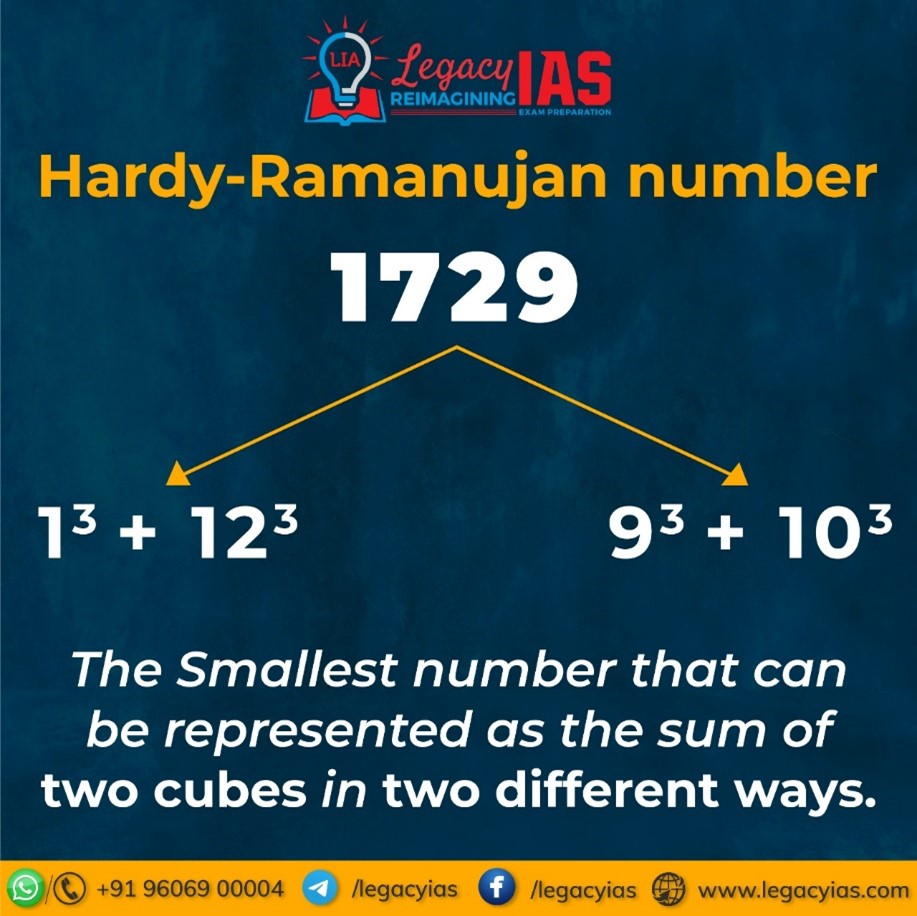
Hardy-Ramanujan number:
Another famous incident that shows Ramanujan’s love for numbers was when Hardy once met him in the hospital. When Hardy got there, he told Ramanujan that his cab’s number, 1729, was “rather a dull number” and hoped it didn’t turn out to be an unfavorable omen. To this, Ramanujan said, “No, it is a very interesting number. It is the smallest number expressible as a sum of two cubes in two different ways.”